Modeling the hydraulic pump
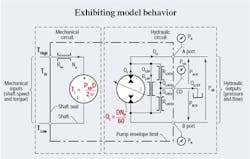
The idea of a set of increasingly detailed mathematical models and their schematic representations was introduced in December 2008’s Motion Control. A schematic characterization of the so-called second and third degree models of a hydraulic pump or motor, shown in the schematic, will be helpful in reviewing some of the basics.
First, the schematic is a bit unconventional because it contains the “ideal” pump/motor along with its frictional and internal loss parameters. The loss parameters, indicated with capital Rs and qualifying subscripts, are laminar, and the losses are laminar in nature. That is, they are directly proportional to speed and pressure, respectively.
Also, a mechanical part of the schematic is formulated to be a hydraulic equivalent circuit that shows the torque relationships. The companion part of the circuit is hydraulic and is used to illustrate the ideal flow generation along with three different internal leakage paths. Together, they completely characterize the inner workings of a positive-displacement hydraulic pump, motor, or both.
Defining the variables
Before proceeding, we need to grasp the physical significance of the various algebraic symbols and their schematic counterparts. Therefore, following is a summary of nomenclature:
• The A port is a notational invention made solely for the purposes of model presentation. It is not part of any standardized notation for pumps or motors. It merely serves to distinguish one power port from the other.
• The B port is also an invention and represents the other pump power input/ output port.
• CD is the case drain port. • I indicates that the schematic element is ideal; that is, without losses of any kind.
• NP is rotational shaft speed, input in the pumping scenario, and output in the motoring scenario.
• PA is gauge pressure as measured at the A port of the pump/motor.
• PAB is differential pressure as measured between the A port and B port of the pump/motor, with the A port pressure assumed to be higher than the B port pressure.
• PB is gauge pressure as measured at the B port of the pump/motor.
• PACD is the differential pressure as measured between the A port and the case drain port of the pump/motor, with the A port pressure assumed to be higher.
• PBCD is the differential pressure as measured between the B port and the case drain port of the pump/motor, with B port pressure assumed to be higher.
• PCD is gauge pressure as measured at CD of the pump/motor (case drain pressure).
• QI is ideal flow, that is, the flow from the mathematical ideal pump/ motor symbol with direction consistent with being a pump versus a motor characterization.
• QLACD is internal leakage flow that makes its way from the A port connection (the assumed high pressure pump port) and from the case drain port.
• QLBCD is the internal leakage flow that makes its way from the B port connection (the assumed low pressure pump port) and from the case drain port.
• QLCDT is total internal leakage flow from the case drain port.
• QLPP is the internal leakage flow that makes its way from the A port (assumed high pressure) to the B port (port-to-port) and ends up being recirculated within the pump.
• RFW is the so-called friction and windage coefficient, or mechanical opposition to rotational speed, in units of torque/rotational speed. It accounts for the mechanical losses within the pump and is caused by both mechanical and hydraulic friction effects.
• RLACD is the linearized, laminar internal leakage coefficient carrying leakage from the A port to the case drain port in units of pressure/flow rate.
• RLBCD is the linearized, laminar internal leakage coefficient carrying leakage from the B port to the case drain port in units of pressure/flow rate.
• RLPP is the linearized, laminar internal leakage coefficient carrying leakage from the A port to the B port (port-to-port) in units of pressure/ flow rate.
• TI is the ideal torque created by the Torque generator, and it represents the torque caused by the differential hydraulic pressure between the A port and B port. It accounts for the fact, for example, more torsional effort is required to rotate a pump shaft when there is a higher pressure at the pump outlet port.
• Tin is the total input torque supplied by a prime mover (pumping scenario) that must overcome the frictional losses caused by the torque loss coefficient, RFW, interacting with the shaft speed, NP.
Some features of the model
First, the schematic of the second degree model will suffice for either a pump or a motor. However, the drawing is annotated to indicate the pumping mode. The interpretations are basic: the mechanical schematic indicates that the shaft speed is entering the higher torque input terminal. Furthermore, inside the mechanical circuit, the torque generator applies a torque that opposes, or works against the speed, attempting to reduce the speed.
This is consistent with the mechanical actions of a pump. In a motor, the torque generator would be “pushing” speed out the high torque terminal. In addition, on the hydraulic side of the schematic, flow leaves the ideal pumping symbol and leaves the assumed high pressure output port. This, too, is consistent with hydraulic pumping action.
Second, the mechanical circuit is depicted as a “hydraulic equivalent” circuit, meaning that torque behaves in a manner analogous to hydraulic pressure, and shaft rotational speed behaves like hydraulic flow. The friction and windage loss coefficient, RFW, behaves like a restriction, so it causes a torque loss. The mechanical circuit has but one equation, which sums the torques, with speed the same throughout. That is, the input torque, Tin, is the sum of the torque frictional loss plus the torque needed to overcome the torque generator, TI.
Third, the pump envelope limit can be interpreted as being the case of the pump or motor. Under predictable conditions, for example, when the case drain port is blocked, internal leakage will pressurize the case.
Mechanically, the shaft must go through the case in order to motivate the internal parts. Therefore, the shaft must have a seal — a dynamic seal because of the relative motion between the rotating shaft and the stationary case. The location of the seal can be visualized at the THigh and TLow mechanical ports.
These two ports are artifices, arising because the decision has been made to characterize the mechanical aspects of the pump/motor as hydraulic equivalents in schematic, rather than pictorial form. I urge readers to view pictorial drawings and photographs of pumps and motors in order to correlate the artificial analytical schematic with the actual hardware.
Fourth, note that pump output ports are labelled A and B, notations that do not apply to any standard pump symbols. It is an artifice used merely to assist in both modeling and schematic clarifications.
For example, the port identifications can be used to indicate the socalled phasing of the pump. That is, we can say, “When the shaft is turning clockwise viewed from the shaft end, and the displacement is positive, flow will issue from the A port.” This information is vital to proper simulation because it will alter the nature of any feedback loops and create a simulated positive feedback when negative feedback was intended. Any simulation will be in a runaway mode and the simulation will crash, analytically speaking.
Fifth, whether pumping or motoring, the model can accurately characterize those conditions where the transition takes place. For instance, in a hydrostatic transmission propulsion system, when a vehicle is going uphill, the pump unit is pumping and the motor unit is motoring. When going downhill, the motor switches to pumping mode (mechanical power is entering the motor shaft) while the pump goes into motoring mode, sending power into the prime mover or braking system. This well-known phenomenon can be observed with the model in its form depicted in the schematic. The A- and B-port markings help in deciphering the results of model usage in either steady state or in a dynamic simulation.
Sixth, pumps and motors can be interconnected to form systems, for example, as with a hydrostatic transmission. Some examples will be covered in future issues. The connections are intuitive and, therefore, easy to sketch. More importantly, though, with the loss elements exhibited in the model schematics, many implications of the interconnections can be studied in detail and with reasonable accuracy. Model usage and interconnections will be covered next month.
Jack L. Johnson, P. E., is an electrohydraulic specialist, former director for the Fluid Power Institute at the Milwaukee School of Engineering, and technical consultant with extensive experience in hydraulics and closed-loop electrohydraulic motion and pressure control. He has authored numerous textbooks, which can be purchased at the H&P Bookstore on our website.
Learn more about servo and proportional electrohydraulics
The Designers’ Handbook for Electrohydraulic Servo and Proportional Systems covers electrohydraulic system design, motion control, and system analysis by teaching applications principles and reviewing practical problems from the real world instead of endlessly discussing complicated theories. It has been written for the practicing applications engineer and technician. It features:
• everything from fundamental hydraulic circuit analysis to dynamic testing of motion control systems,
• more than 500 pages of useful formulas and dozens of fully worked examples, and
• 20 chapters covering hundreds of topics, including components, systems, circuit analysis, modeling, servomechanisms and control, simulation, and testing.
The book sells for $149.95, plus $5 shipping and handling from the H&P Bookstore. To order, visit www.HydraulicsPneumatics.com and click on the Bookstore button. Then scroll down to the hyperlink to download a PDF order form. Fill out the form, fax it to the number indicated, and your order will be shipped promptly.
You can also order by phone by calling (216) 931-9427. Or your can order by e-mailing credit card information to [email protected].