Save Energy by Recycling Air

Although pneumatics technology provides a clean, high-power, low-cost means of implementing factory automation, the compressed-air costs associated with pneumatic systems are notoriously high—often accounting for up to 30% or more of the power costs at a plant. In the U.S. alone, it has been estimated that the energy used to compress air exceeds a half-quadrillion BTUs per year.

Nexmatix LLC has developed an innovative directional control-valve technology that provides a plug-and-play replacement for a standard directional control valve. Typically, it yields a 25% savings in compressed-air costs without compromising system function. The intent of this article is to illustrate cost savings provided by Nexmatix technology in a typical pneumatic actuation application.

The Cost of Compressed Air

One of the primary contributors to the high cost of compressed air is the relative low efficiency of compressed-air systems, which are typically characterized by efficiencies from electrical input power to compressed air outlet power of 10% to 14%. The nature of these inefficiencies is not always well understood, and as such the authors use this section to derive and explain the electricity costs associated with operation of a compressed-air system.

In assessing the costs associated with a pneumatic system or component, it is most convenient to compute costs as a function of the compressor outlet air flow. The relation between the electrical input power to a compressor and the pneumatic power at the output of the compressor is:

P_o = η_m × η_c × P_i (1)

where:
P_o is power out (available pneumatic power);
P_i is power in (electric power supplied to compressor);
η_m is motor efficiency from electrical power input to rotational mechanical shaft power
output (typically between 85% and 95%); and
η_c is compressor efficiency from rotational mechanical shaft power input to constant pressure outlet air power (typically between 10% and 18% for positive displacement compressors).

The available pneumatic power assumes that the pneumatic component using this power will not extract heat as work from the compressed air. Alternately stated, the temperature of the compressed air, when used by the pneumatic component, is not significantly higher than the ambient temperature of the system. In other words, after it has been compressed, the air performs work in the same manner as does a hydraulic component. This is consistent with the manner in which pneumatic components perform work in an automation environment. Given this assumption, the power that can be utilized from constant pressure and volumetric flow is:

P_o = (p_o – p_i) Q_o (2)

where:
p_o is absolute pressure at the outlet of the compressor;
p_i is absolute pressure at the inlet of the compressor; and
Q_o is volumetric flow rate at the outlet of the compressor.

Defining outlet gauge pressure, p_g, as (p_o – p_i), Equation 2 can also be written as:

dW_o/dt = p_g× dv_o/dt (3)

where:
W_o is work output;
v_o is volume output; and
t is time.

Nexmatix valves are designed as drop-in replacements for valves conforming to ISO 15407 and 5599. They are also color-coded to make it easy to identify spool configuration from a distance. Each valve also contains a QR code so a smartphone can be used to pull detailed information about any individual valve.

Assuming steady-state conditions, both sides of Equation 3 can be integrated to yield:

W_o = p_g × v_o (4)

Likewise, both sides of Equation 1 can be integrated to provide a relationship between input energy and output work:

W_o = η_m × η_c × E_i (5)

where:
E_i is input energy.

Combining Equations 4 and 5 and solving for input energy results in:

E_i = p_g × p_o/η_m × η_c (6)

The following relationship is used to introduce cost to the analysis:

C = α × E_i/(1– β) (7)

where:
C is a cost of running the compressor system, $;
α is cost of electrical power, $/J); and
β is the dimensionless cost factor of running the compressor system (typically 0.2 to 0.3).

The cost factor, β, is site-specific and typically includes maintenance, capital depreciation, water- cooling costs, and other overhead associated with the system.

Substituting Equation 7 into Eq. 6 and solving for cost yields:

C_o = α × p_g × v_o/[ η_m × η_c (1– β)] (8)

or as a cost specific to volume:

Ä_o = α × p_g/[ η_m × η_c (1– β)] (9)

where Ä_o is the cost per volume of compressed air in its compressed (outlet) state.

A conversion factor is applied to put this cost equation into more commonly used units:

Ä_o = 0.0543 × α × p_g/[ η_m × η_c (1– β)] (10)

where:
Ä_o is in $/MCF (1 Mft³ = 1,000 ft³);
α is in $/kW-hr; and
p_g is gauge pressure, psi.

This cost calculation of air in its compressed state is a critical distinction from the compressor’s inlet air. Although computing the cost of air relative to the outlet flow is most direct, it also requires knowledge of the outlet pressure for a given application. To remove dependence on the outlet pressure, the cost can instead be transformed, using the ideal gas law, to an equivalent volume of air at the inlet (i.e., at standard conditions). To determine the cost of compressed air relative to the inlet flow, simply apply the ideal gas law.

For a given mass of gas at a given temperature effects, the ideal gas law simplifies to:

p_o × v_o = p_i × v_i ; v_o = p_i v_i/p_o (11)

The temperature of a compressed gas used for pneumatic actuation is typically at a standard temperature, as is the inlet air. Substituting Equation 11 into Equation 8, dividing by v_i yields the cost of compressed air per inlet volume. Applying the unit conversion factor:

Ä_i = 0.0543 × α p_g/[η_m η_c (1– β)] × p_i/p_o (12)

Or more simply:

Ä_i = p_i/p_o × Ä_o (13)

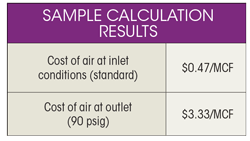
Sample Calculation

This calculation is intended to provide an example of the average cost of compressed air in a typical U.S. industrial setting using real-world data. Assumptions for this scenario include standard inlet conditions (14.7 psia and 20°C) and an outlet pressure of 90 psig. The remaining average input variables were gathered from various sources, including compressor manufacturers’ curves and user data:

Motor efficiency, η_m , is 90%;
Compressor efficiency, η_c is 15%;
Cost of electrical power, α is $0.07/kW-hr; and
Compressor system cost factor, β is 24%.

Using Equations 10 and 13, the cost per MCF of air at the inlet and outlet is shown in Table 1.

Published data for the cost of compressed air ranges from $0.18/MCF to $0.66/MCF. This range is the cost for the inlet flow. The outlet flow cost is typically seven to eight times higher due to the same mass of air being compressed into a smaller volume.

Experimental Verification

A simple test to illustrate and verify this cost analysis was designed and conducted in our laboratory. A power meter was used to measure the total energy delivered to a 2-hp reciprocating air compressor while it supplied 90 psig air to cycle a double-acting cylinder through a 5-port, 3-position valve. The total calculated displaced volume per cylinder stroke was 5.7 in.³. After 5,000 cycles of cylinder actuation, the energy consumed by the compressor was measured as 1.58 kW-hr. The total volume of compressed air displaced was:

5.7 in.³/stroke × 1 ft³/1728 in.³ × 2 strokes/cycle × 5,000 cycles = 33 ft³

Assuming $0.07/kW-hr as the cost of power, the volume-specific cost of the compressed (outlet) air is:

1.58 kW-hr × 0.07 $/kW-hr ×1/33 ft³ ×1,000 ft³/Mft³ = $3.35/Mft³

At standard (inlet) conditions (Equation 13):

$3.35/Mft³ × 14.7/(90+14.7) = $0.47/Mft³

Although this value is in close agreement with the theoretical outlet value from Table 1, it does not include a cost factor, β, which, if included, would increase the $/MCF further. Note that the 2-hp laboratory compressor likely has a lower efficiency than what was assumed for the industrial compressor of the sample calculation, thus resulting in a somewhat higher cost than estimated in Table 1.

ORNL Confirms Energy Efficiency of Valves

The Oak Ridge National Laboratory has published a report on the feasibility and testing of a lightweight, energy-efficient, additive-manufactured pneumatic control valve. Results confirmed that the valves “are as energy efficient as stated.” The report went on to say, “measuring air consumption per work completed, the Nexmatix valve was as much as 85% better than the Festo valve” to which it was compared.

Unconventional Pneumatic Technology

Proprietary solenoid valve technology from Nexmatix recycles an average of 30% of the air otherwise exhausted to the atmosphere, thus reducing air consumption without compromising actuator function. The valves are a plug-and-play replacement for standard off-the-shelf ISO valves typically used for controlling air cylinders. Reducing the air consumption results in lower electricity and compressor maintenance costs. The technology can be applied to most popular 5-port/3-position and 5-port/2-position valve lines with negligible cost difference.

The technology works by briefly connecting the valve’s two cylinder (outlet) ports during each stroke. As the valve cycles, the pressurized cylinder port connects to the opposing (unpressurized) cylinder port, recycling the compressed air from one end of the cylinder to the other. This effectively precharges the depressurized end before it is connected to supply, saving on average 20% to 40% of the compressed air.

The two ends in fluid communication are allowed to briefly dwell (equalize) before venting the remaining spent air from the first cylinder port. This connection occurs on the order of tens of milliseconds and is transparent to the user. For a three-position valve, typical center-position behavior is maintained during power down or loss of pressure.

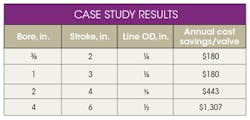
Table 2 summarizes a variety of typical cylinders used in industrial environments and the respective annual cost savings that can be expected by incorporating Nexmatix directional control valves to actuate these cylinders. These examples apply the same parameters used for the calculations described above. The examples assume two-shift operation, cycling 60 times per minute, and air-line lengths of 20 ft.